Simplified Hartree-Fock Computations on Second-Row Atoms

1/3

Modern computational quantum chemistry has developed largely from applications of the Hartree–Fock method to atoms and molecules [1–3]. A simple representation of a many-electron atom is given by a Slater determinant constructed from

occupied spin-orbitals:

[more]

Contributed by: S. M. Blinder

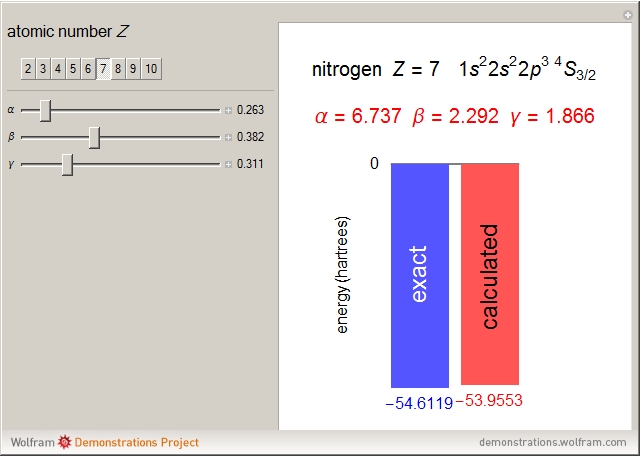
Following are results for optimized functions ψ_1 s, ψ_2 s and ψ_2 p. For comparison we also include results from the best Hartree-Fock computations and the exact atomic ground-state energies.

References

[1] S. M. Blinder, "Simplified Hartree-Fock Computations on Second-Row Atoms," https://arxiv.org/abs/2105.07018

[2] A. Szabo and N. S. Ostlund, Modern Quantum Chemistry: Introduction to Advanced Electronic Structure Theory, Mineola, NY: Dover, 1996.

[3] S. M. Blinder, "Introduction to the Hartree–Fock Method," in Mathematical Physics in Theoretical Chemistry (S. M. Blinder and J. E. House, eds.), Elsevier, 2018.

S. M. Blinder

"Simplified Hartree-Fock Computations on Second-Row Atoms"
http://demonstrations.wolfram.com/SimplifiedHartreeFockComputationsOnSecondRowAtoms/
Wolfram Demonstrations Project
Published: October 4, 2017

https://demonstrations.wolfram.com/SimplifiedHartreeFockComputationsOnSecondRowAtoms/